Assume we want to benchmark the fair odds of a market, but there is no liquidity at the exchange.
We can estimate the Fair Odds of an event in a Closed (or Complete) market by analysing a bookmakers prices, determining the markup and estimating bias.
A complete betting market is a market that contains prices for every eventuality. For example in 1×2 (Match Odds) we can bet on Home Team, Draw and Away Team. This market is complete as one of those three eventualities has to win. An incomplete betting market may be “Christmas Number 1” or “Player to score 3 or more tries”. It is not practical to price up every potential song in existence for Christmas Number 1, nor is it practical to price up the probability of a Prop Forward to score a hat-trick of Tries when he’s never scored a Try in his career.
We can work out the exact margin applied to complete markets. An easy example is any handicap market:
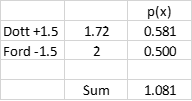
In the Match Handicap market above, Graeme Dott is 1.72 to win with a -1.5 frame handicap, and Tom Ford is 2.00 to win with a +1.5 handicap. There are no other outcomes in this market; either Graeme Dott wins by 2 frames or more and the first bet wins, or any other outcome sees the 2nd bet win. Every outcome is accounted for by these two prices.
We can workout the exact markup used of a complete market by adding together the probabilities of all of the outcomes:
In the market above, we see an overround of 108.1% has been used. In other words, the bookmaker is making 8.1% profit for every £ staked on the market. We can use the figure of 108.1% to estimate the fair prices – the probability of the events without any markup.
Markup – No Bias
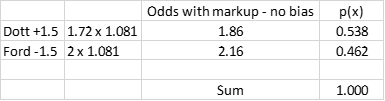
The easiest way to work out estimated Fair Odds is to multiply each of the odds by the markup.
In this example we estimate that the Fair Odds of Dott +1.5 = 1.86 and the price of Ford -1.5 = 2.16 before any margin has been applied. This is a approach assumes there is no bias applied.
Markup – Bias
Bookmakers will often apply bias to one side of the market to optimise their profit. For example, if a bookmaker knows that Ford is a crowd favourite and Dott is a villain, the bookmaker may expect more money is going to be bet on Ford, and therefore the bookmaker will apply more margin to Ford and less to Dott. A similar bias exists in many other areas of gambling where heavy favourites are not bet to the same liability as longshot outsiders. A punter may be £5 at 100/1 on a horse, but wouldn’t dream of betting £1000 at ½ on a competitor, yet both bets carry the same liability for the bookmaker. To account for this, bookmakers will apply fav-longshot bias and will apply more margin to outsiders than favourites. Evidence of this can be seen on horse racing markets where the favourite of a horse race is often a close arb at top price, yet a horse at top price of 66/1 at the bookmaker may be 300 or higher on the exchange.
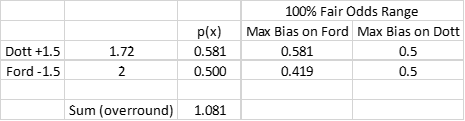
There are a number of ways to account for bias, but all must fall within the range of markup determined:
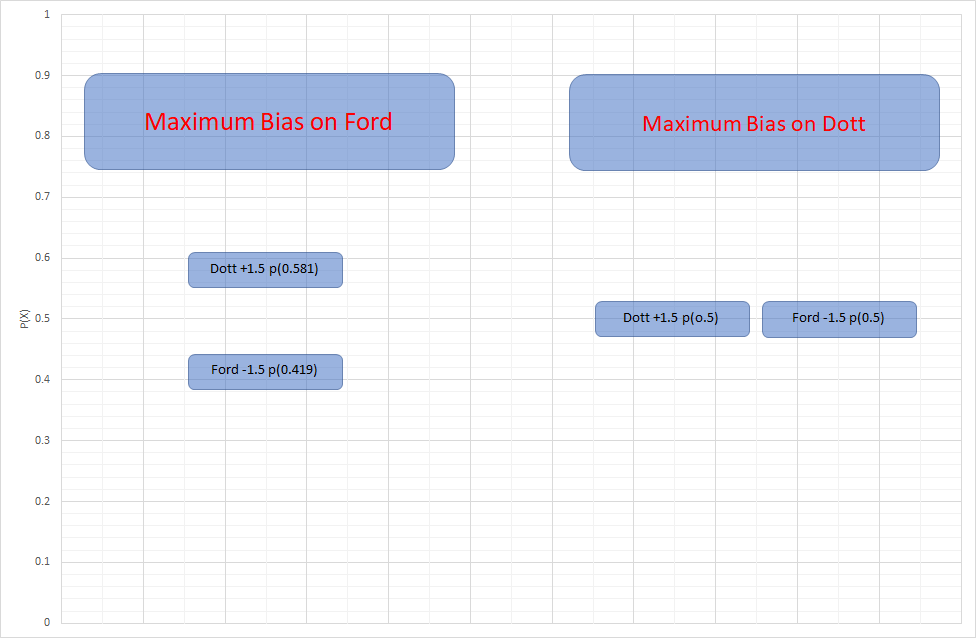
In the example above, there is 8.1% markup to play with. A fair reflection of the market needs to equal 100%. Therefore the maximum bias on Ford would be his bookmaker price p(0.5) – 0.81 = 0.419. This assumes zero bias towards the favourite, Dott. Conversely, maximum bias on Dott would be his bookmaker price p(0.581) – 0.81 = 0.5, assuming zero bias on Ford.
It is an inexact science to estimate which side to apply bias. Whilst we never assume to understand the exact bias applied by the bookmaker, we can confidently determine the range (0.581,0.419 to 0.5,0.5) within which the fair odds should have originated. Some common sense can be used. If a crowd favourite is playing an unknown, we can make a guess that the majority of the punters money is with the favourite. In a market such as Point Spread in NFL, we can assume that sharp syndicate money shapes it within an inch of its life, and therefore bias could be marginal.
Tips:
- Use a range of bookmakers to determine the “average” price of a set of prices in a market.
- Step back from the event and think it there is a reason for the market to have a volume of on one particular outcome. It is likely that this outcome will be the focus of bias at the bookmaker.